37 PERCENT RULE - Why knowing this rule will give you exclusive power and ultimate satisfaction?

Whether you're choosing a candidate or a spouse or a storefront, this math-based trick aka 37 percent rule can help you pick well.

May 15, 2022

When do you give up on information gathering and decide you have enough data to make the following example of choices?

How many people should you date before deciding to ‘settle down’ and get married?
How many interviewees should we look at before deciding on "The One"?
How many flats should I look upon before saying "Yes" to one of the offers?
How many hotels should I look before deciding on the one to settle into?
How many internships should you do before deciding on a job offer?
How many careers should you try out before you figure out which your favourite is?

Above are the classic examples of a situation where you feel:

Spend too long probing your options and you fall prey to analysis paralysis and let promising opportunities go by. (Overly Exploratory)
Close out the process too quickly and you risk regretting options you never considered. (Overly Exploitative)

The answer to above situations is to follow a single mathematically derived magic number 37, no matter what domain your decision falls in.

“Be prepared to immediately commit to the first thing you see that's better than what you saw in that first 37%.” - Brian Christian

MAIN IDEA

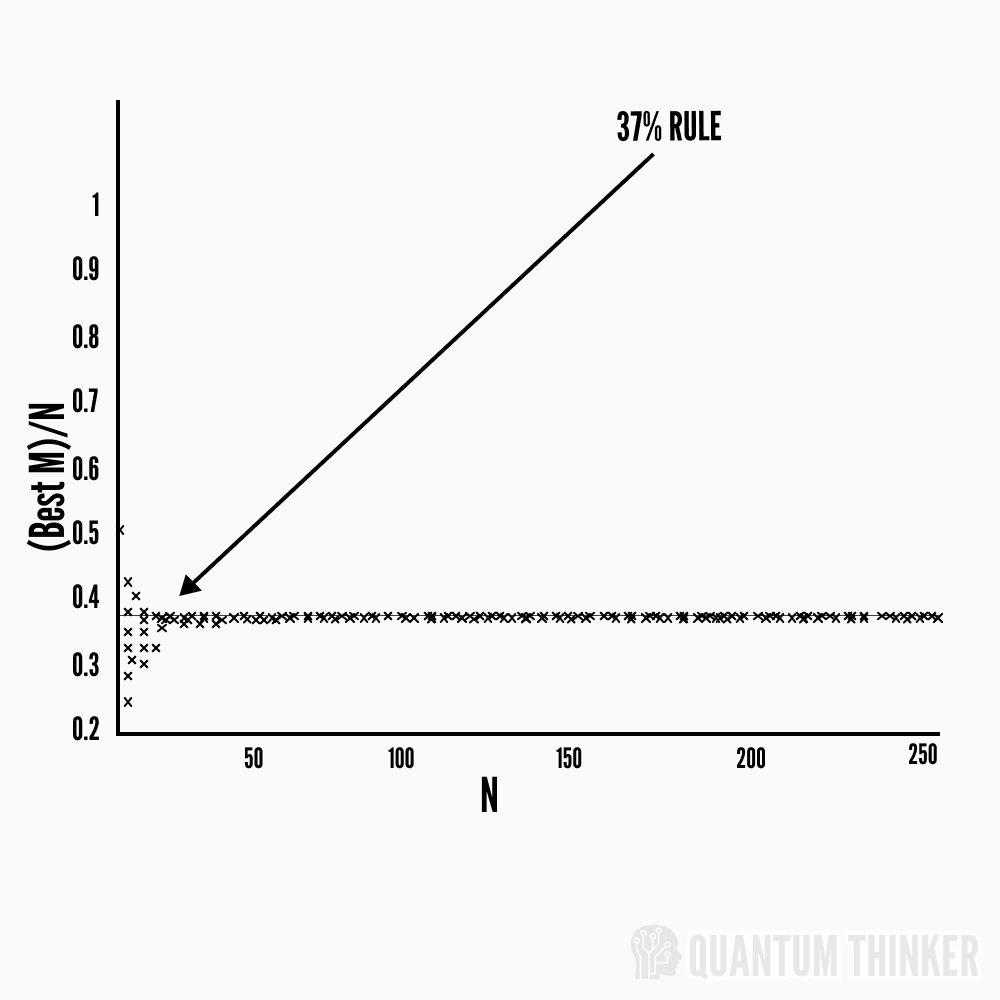
The 37 percent rule comes from ‘optimal stopping’ theory in mathematics, which determines the optimal time to take a particular action in order to maximize reward and minimize cost (aka, the best time to stop seeking more options and pull the trigger). According to mathematicians, that point is right after you’ve seen or explored the first 37% of your options.

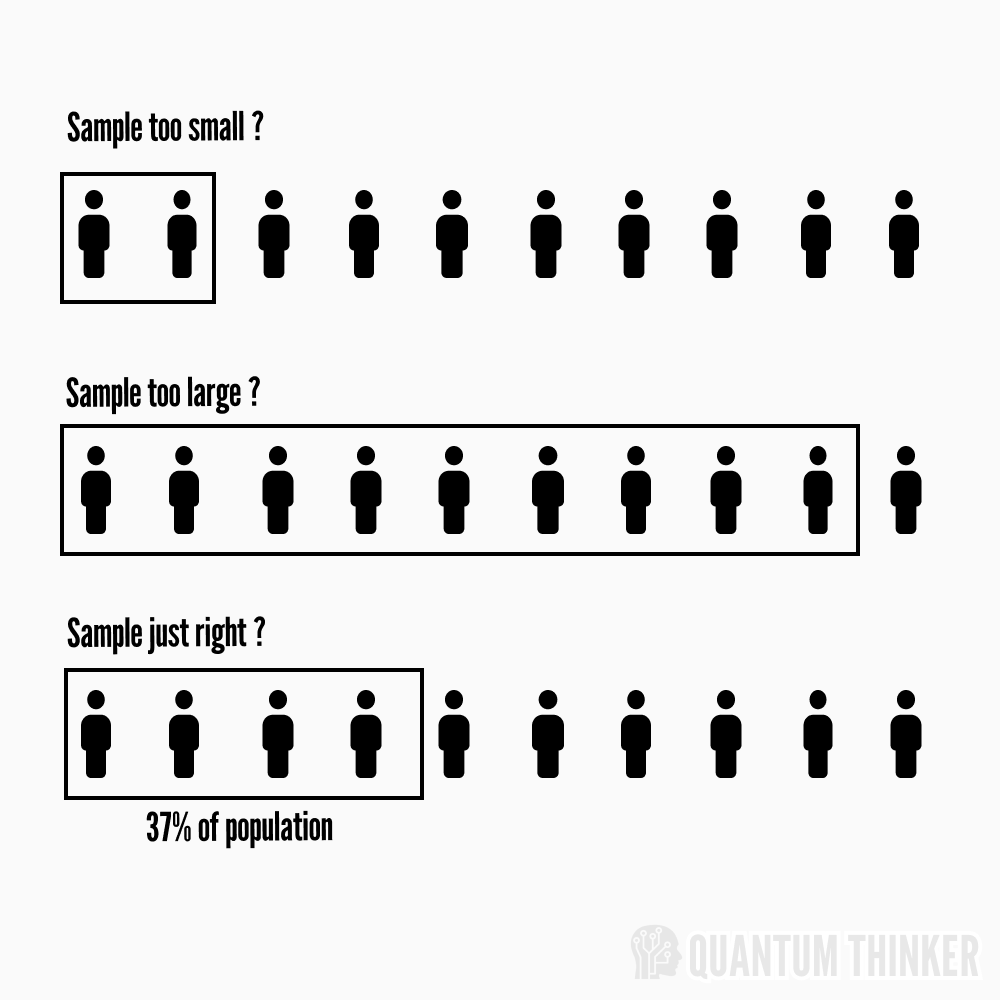
Of course, you need to come up with your total universe first, to calculate what 37% of your options will be. You can do that either by setting a maximum cap or a time-based deadline.

Let’s consider the example:

If you’re hunting for a new car, and decide you’d like to see 10 cars before making a decision, you should plan to see the first 3-4 with zero intention of buying them. After exploring those, your exploratory period has reached a point of diminishing returns, and the next car that is better than those initial three is the keeper.

Although it is true that matters of the heart are not rational, this rule merely increases the likelihood. It does not ensure achievement. Therefore, don't allow arbitrary rules get in the way if you suddenly know you've discovered the one when lightning strikes or the angels sing.

However, even if applying the 37 percent rule blindly in every situation is not a good idea, that doesn't mean it is useless or ineffective. Understanding that it's normally best to spend the first third of your decision-making process acquiring facts will help you avoid FOMO and analysis paralysis. You may find it easier to summon the guts to act when you are aware that you should hire the first applicant who outperforms the possibilities you have previously considered.

The secret to making outstanding decisions is striking the right balance between consideration and decisiveness, and the 37 percent rule can help you achieve just that.

Of course, when it comes to large financial decisions and matters of the heart, this rule doesn’t account for feelings, “gut instincts,” instant chemistry, and the power of recommendation. (E.g., when there is a very attractive, hilarious person sitting across from your very single self who was vetted and suggested by a close friend, even if they are the first date you’ve gone on in a year.)

TECHNICAL (1/e Stopping Rule)

This oddly precise recommendation actually has a formula behind it and mathematicians insist this rule gives you the highest probability of making the optimum choice. If you're into math, it's actually 1/e (1 over e, where 'e' is the basis of the natural logarithm), which comes out to 0.368, or 36.8 percent or 37% as the number of choices increases.

PSYCHOLOGICAL TEST on Exploit or Explore Conundrum

Mathematics provides the best solution to the “optimal halting problem.” But there’s just one big issue with it: Humans are not rational probability-crunching machines. In fact, the opposite is usually true. We’re beautifully, infuriatingly, creatively, and messily chaotic. So, it falls on psychology to tell us about how we actually behave.

In psychology and economics, there is what’s known as a “explore/exploit” trade-off. This asks whether you should go with a guaranteed “win” (the exploit) or risk going somewhere else for an unknown outcome (explore). The degree to which someone will explore, or exploit will depend on a host of factors, and it ties in with how curious or risk-seeking we are.

According to Addicott's research, which was published in Nature, being excessively explorative or too exploitative puts us at a disadvantage. A person who investigates excessively runs the danger of becoming a “jack of all trades, master of none,” while a person who exploits excessively “may foster habit building.” Over-exploiters lose motivation, grow bored, and get caught in a rut. Over-explorers lack knowledge and never fully immerse themselves in anything. The best behaviors, according to Addicott and his team, “occur at a point of equilibrium between the two.”

Of course, different people are more explorative or exploitative at different times. Teenagers and entrepreneurs tend to explore more. Adults and managers exploit more. Try testing yourself with these three questions, to see where you fall:

If you are visiting a city, you know vaguely well, will you go to a restaurant you know is nice, or will you try somewhere new?
If I tell you a gambling machine has a payout of $50, will you stay and play or explore to see if others have a bigger payout?
When you’re playing a game, do you tend to stick to the same tactics or mix it up each time?

“True optimization is the revolutionary contribution of modern research to decision processes.” - George Dantzig

1) SECRETARY PROBLEM OR HIRING PROBLEM

A historical problem that derived this rule called "Secretary problem" at around 1960.

Let’s suppose you want to hire a secretary. You want to find the best person for the job, but you won’t know how good someone is until you interview them. At that point, you can choose to hire them (a permanent decision) or to move on and interview someone else. The only catch is that once you’ve rejected someone, you’re not allowed to go back and hire them.

How do you maximise your chances of finding the right person?

If you're hiring a secretary and plan to interview 10 candidates, let the first three go by. Then choose the next person who beats that first bunch.

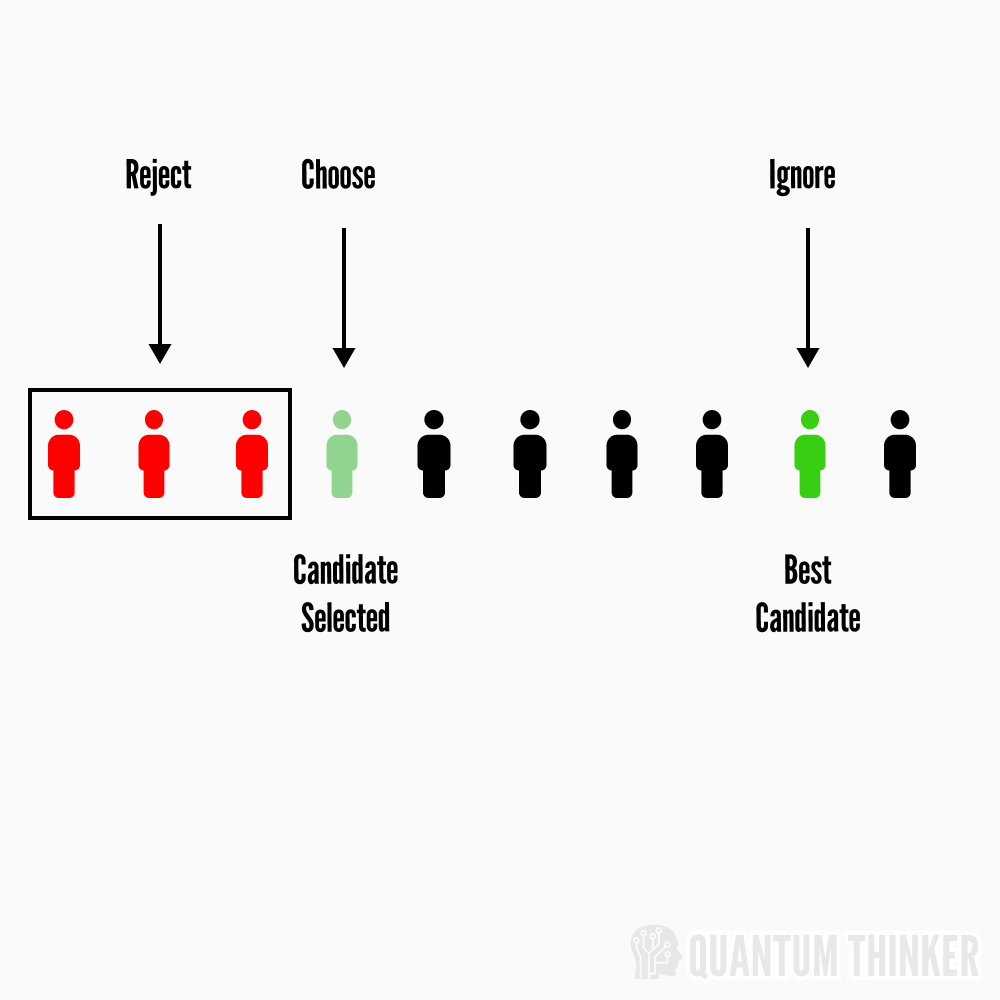
Basically, if you knew you were interviewing 100 potential secretaries, you’d interview and reject the first 37, and you’d keep in mind who your best-so-far candidate was as a benchmark. From interview 38 onwards, you’d immediately hire the person better than your best-so-far candidate. This is apparently how you maximise your chances of hiring the best overall candidate.

Of course, real-world situations don’t always conform so neatly to math theories. Not knowing how many applicants you’ll get for an open position would make it hard to know when to stop interviewing and start hiring. Still, imposing some structure and limits to a process that can too often drag on can only benefit you — and will kill your FOMO.

2) MARRIAGE PROBLEM

So, let's think over it for some time, what essentially the solution says is that if you have a consideration set of around 10 girls before you decide which one to marry, you should not commit to anyone, until you have looked through at least 4 of them. Post which you should be ready to commit to anyone who is better than all previous ones.

If you're hoping to be settled down by 40 and start dating in high school, take the first third of your dating life -- up to around age 25 -- to casually check out your options.

The magical insight is from Logan Ury’s book (How to die not alone) is that we don’t need to think about it in terms of numbers - we can think about it in terms of time.

It’s very hard to know how many people we could end up feasibly dating, but it’s easy enough to estimate how much time we want to spend dating.

If for example, I’m open to dating between age 18-40 (and assuming there’s no radical change in the number of people I’m getting to know each year), the 37% rule says that when I hit the age of 26, I should marry the next best person.

3) REAL ESTATE PROBLEM

In his book "Algorithms to Live By: The Computer Science of Human Decisions", Brian Christian applies the 37% rule to assist Macy in finding the ideal apartment to rent. In his words: "Assuming you want the highest odds of acquiring the best apartment, spend 37% of your apartment search, or the first third (eleven days, if you've allotted yourself a month for the search), noncommittally examining choices. You're just calibrating; leave the check book at home. But following that, be ready to immediately commit—deposit included—to the first location you find that surpasses all you've already viewed. This is more than just a naturally satisfying middle ground between looking and leaping. It is the demonstrably best course of action.

CONCLUSION

Following this rule will save you from getting unnecessarily mired in information gathering and data analysis, get you into action, and maximize your probability of success.

While it won’t apply to every situation, and you shouldn’t discard what are clearly superior options simply because of a mathematical theory, if you have a tendency to make hasty but regrettable decisions, or spend too long investigating all your options, it’s a helpful rule of thumb. The next time you’re faced with competing choices, remember: Roughly the first third of your decision-making process should be information gathering, after which time, selecting the next great option you encounter is optimal.

I am sure that there are many who are already aware of this, but still publishing a post to reach out to as many as possible who don't know about Optimal Stopping problem and its solution and are going through their lives trying to reinvent the wheel.

TLDR:

When trying to pick the best among many options, how many samples should you try before you commit? This is known as the optimal stopping problem.
Mathematicians advise us to eliminate the top 37% of any possibilities in order to increase the likelihood of the best result.
People typically "explore" or "exploit" more, depending on the psychology. Unfortunately, relationships are messier than probability would suggest.

“Life is Quicker Than a Blink of an Eye” - Jimi Hendrix

“The best kinds of failures are quick, cheap, and early, leaving you plenty of time and resources to learn from the experiment and iterate your ideas.” - Tom Kelley

“Better a good decision quickly than the best decision too late.” - Harold Geneen

Mathematical proof of the 37% Rule: https://plus.maths.org/content/solution-optimal-stopping-problem

Thanks for reading…

3M Notions

Discussion about this post